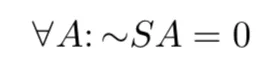C17 丘奇、图灵、塔斯基及别的人
领读人:松乔
时间:2022年6月8日?
分享会录屏链接: https://pan.baidu.com/s/1hFmAX9qW6f8Nk3MiapoPXA?pwd=r59j
密码: r59j
--来自百度网盘超级会员v5的分享
的确该赞美螃蟹 The Magnificrab, Indeed
爬山、喝茶、演奏音乐
Escher 1930
概要: 乌龟和阿基里斯一起登山,据说山顶有极好的茶馆,两人赞美螃蟹,乌龟提到一个螃蟹的印度仰慕者,是一个印度人,把螃蟹当成了数学家。印度人衍奴玛拉用自己的方法推算出数学定理,比如说到了有意思的1729数字(后文解释)等。螃蟹说结果都对但是不知道他是如何发现这些结果的,某种东方式的洞察力,螃蟹觉得是怪诞的神秘学解释,但是乌龟不同意,认为数学研究不可能与已知的方法完全不同 - 引出丘奇-图灵论题
音乐部分,螃蟹看乐谱演奏音乐,而他的乐谱在阿基里斯看来就是数论语句
皮亚诺第三首场地前奏曲:
0不是任何自然数的后继
阿基里斯开始用数论作曲给螃蟹演奏,然后两人讨论如何改编曲子更好,然后讨论到是否改变一些小细节,曲子还是美的。
螃蟹:我敢说不存在什么现成的规则,可以用来描述究竟是什么决定了一支曲子是不是美,而且永远也不会有这样的规则。美感只能存在于有意识的心灵之中,这种心灵靠生活经验的积累所达到的深度,超越了一切由一组规则所能做到的解释。
两人同意对美的解释也可以用于真理概念,像数学与音乐
阿基里斯然后再不断的试探螃蟹是否可以演奏不同的曲子,来了解如何判断曲子是否是美的
印子中螃蟹似乎天生有对于美的直觉和解释,是一种超越规则的能力,跟印度人解数学题类似,而阿基里斯不同意,想要通过对数论结构的探究找到螃蟹演奏音乐美感的原因,引出下文
Formal and Informal Systems 形式和非形式系统
思维/大脑是由简单的形式系统(formal, simple systems)支持着非形式系统(underly informal systems),外部看起来是非形式化,公开,软件,对应的是形式化,隐蔽和硬件的机器与神经元,规则,可以说大脑是某种数学的对象。
数学处理的是简单而优雅的系统(math deals with simple and elegant systems),而人工智能研究与数学研究不同的就是,人工智能尝试的是不断在越来越高的层次上组织,形成一个最上面的非形式系统,而最上面的形式系统我们还没有具体的词汇去表达。
Intuition and the Magnificent Crab 直觉和值得赞美的螃蟹
人工智能(artificial intelligence)也是人工直觉(artificial intuition) 或者人工意象 (artificial imagery),因为人工智能需要有辨别能力,需要辨别能力,可以知道什么重要和什么不重要,(简洁和对美的感受力),那这些直觉哪里来的呢?
举例说明
"我从报上读到。。
“你读报来着?你有眼睛?至少有一只?至少读报的时候有一只?"
有智慧的螃蟹,似乎可以辨别什么美,什么不美,阿基里斯认为它的方法是通过数学,分为真的和假的数论,但是螃蟹并不承认。这而且好像直接违背了丘奇定理和 Tarski-Church-Turing Theorem
丘奇定理:church's theorem, noinfallible method for telling theorems of TNT from nontheorems
Tarski-Church-Turing Theorem, no infallible method for telling true from false statements of number theory
The Church-Turing Thesis 丘奇图灵论题
理解两个定理前,现描述一下作为它们基础的思想,叫丘奇论题,非常重要,而且像泡茶一样有不同的浓度以及许多不同的表现形式
- 同义反复形式:数学问题只能通过数学推演来解决。 innocent and pointless/tautological version: math problem can only be solved only by doing math
- 标准形式: 假设有一种方法,一个有感知能力的生物可以根据这种方法逐个把数分成两类。假定这种方法总能在有穷时间内得出答案,而且对于给定的数,这种方法总给出相同的答案。那么:存 在一个有终止的 FlooP 程序(即一般递归函数),它给出的答案恰好与这个有感知能力的生物的方法所 得到的答案一样。standardversion: sentient being: sort numbers in 2 classes, always yields answer within a given time and the same answer, then FlooP can give same answer ( some general recursive function exists)
丘奇图灵论题不是一个可证明的事实,而是关于人脑中所使用的过程的一个假设。the hypothesis is about the proceses which human brains use
大众过程形式The Public-Processes Version
螃蟹对数学有神奇的洞察力,但是它不了解自己的特殊能力,找不到对应的floop的运算,Crab can have a mystical insights into math, but in the dark of his mental abilities and there is no floop counterparts of his mental mechanism (potential for subconscious to taranscend consious and inexpressible in FlooP
- 大众过程形式:如果有可以用语言传达的方法的话,就有对应的floop程序。只界定了大众接受的方法,但是没有断定个人特有的方法,保持开放 Public Processes Version : if the mental process can be communicated reliably from one to another by means of language, then some terminating Floop program exists to derive at the same answer
湿利尼吠萨 拉玛奴衍 Srinivasa Ramanujan
Srinivasa Ramanujan, from TamilNadu, 1887-1920, sent his discoveries to G.H. Hardy, in 1913 came to England 来自泰米尔邦的印度数学家,求教英国的哈代教授,受哈代教授来到英国,至死前两者进行了合作。
拉玛的不寻常的特点包括Extraodinary Characterisitics
- 缺乏严格性,仅仅简单陈述结果说来自自己的模糊直觉,使之神秘化lack of rigor, insist a result from a vague intuitive source, goddess Namagiri inspired him in his dreams
- 某种“东方神秘主义”:他难免出错加强了相信他直觉能力的信念,而且他所在地的各种古怪习俗也给了他某种西方人不可企及的正确性some kind of "oriental" rightness, the fact he could be wrong made him more credible, touching upon truth inaccessible to western minds
- friendship with integers与整数的友谊,两个小故事,一个就是关于上文提到的 1729,是出租马车,是两种不同方式表示称两个数的立方和的最小的数
哈代不相信那些假设,而认为所有的数学家的思维方式应该有统一性 Hardy thinks that all mathematicians think at bottom in the same kind of way
- 哈代版本hardy's version: 本质上来讲,所有的数学家都同构 all mathematicians are isomorphic
但哈代对rama有一种浪漫式的描述 his power of generalisation, a feeling for form and acacpacity for rapid modification of his hypotheses, an characterization of some of the subtlest features of intelligence in general
心算家Idiots Savants
they are not idiots, just lightening speed calculators
这些奇才也是用一样的方式来进行计算,只是比我们快很多,并没有超自然的过程,他们计算能力超过解释自己结果的能力。
同构形式The Isomorphism Version of the Church-Turing Thesis
- 同构形式: 这个心智过程与这个 FlooP 程序在下述意义上同构:在某个层次上,计算机和大脑各 自执行的那些步骤之间存在一个对应。 The mental process of the brain and Floop program are isomorphic, correspondence between the steps being carried out in both computer and brain
对现实世界的知识的表示 Representation of Knowledge and the Real World
数论是小和清晰的,但是现实世界非常复杂,real world much more complex than number theory
难以撇出的过程Processes that are not so skimmable
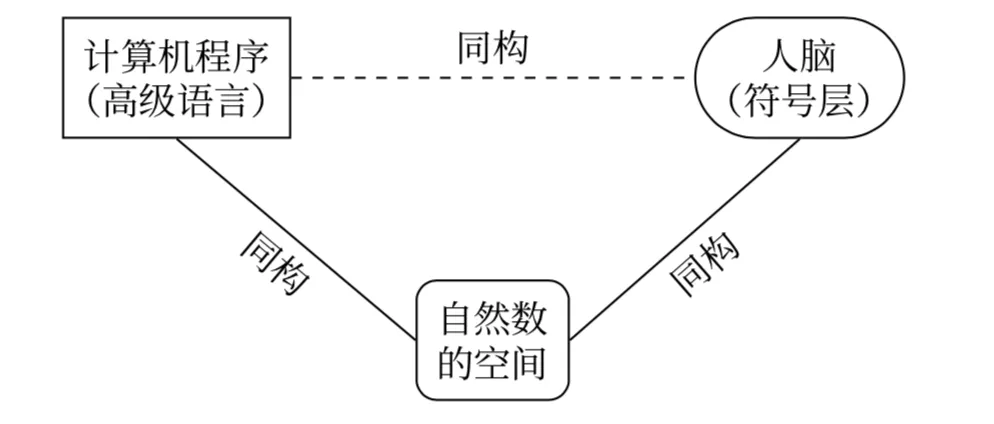
大脑是一个很特别的形式系统 brain look like very peculia formal system
最底层:神经元,原始的元素,神经元发射bottom level neural level, the rulse operate and change the state, neural firings
顶层:有意义的解释,从大团的神经元活动形成符号进行对真实世界的解释。 top level: meaningful interpretation, mapping from the large clouds of neural activity which we call symbols onto the real world,
类似于哥德尔的构造,在哥德尔的构造中,高层意义是从较低的层次上导出的
而在大脑中,在神经元层面的活动仅仅是实现较高层次的基质,像是计算器里的晶体管。
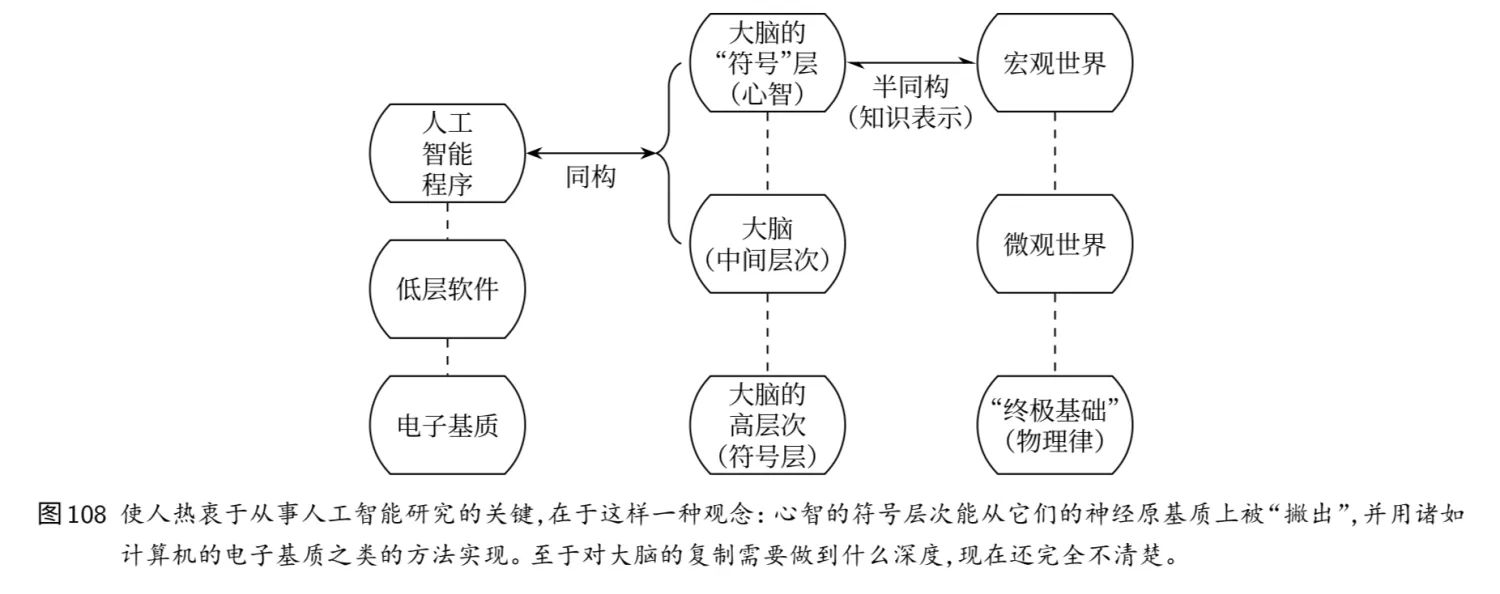
所以不能仅仅是将最高层挑出来然后进行一个高层的同构,如果要做大脑思维过程的镜像程序,需要反应最底层,包括硬件。要对外在世界进行有智慧的解释的话,也需要不同层次的程序,最底层级成为上层的基质,而不是他们直接与外在世界产生直接的连接。(蚂蚁赋格中提出从字母层级去理解一本书会使其成为一个无法描述的无聊的噩梦)
如果仅仅是进行演绎推理deductive reasoning processes,本质上是在一个单一的层次上程序话,所以可以撇出,而包括想象和类比的思维过程就需要基层不同的基质,本质上是不可撇出。创造性开始出现,本质上依赖于某种“不可解释的”底层事物 at this point creativity starts to emerge, creativity intrinsically depens upon certain kinds of uninterpretable lower-level events
简化论的几个信念Articles of Reductionistic Faith
意义用的较高层次出现是有选择余地的。Emergence of a higher level of meaning is optional, but not vice versa, the higher level must be derived from properties of a lower one
- 微观形式Church-Turing Thesis, Microscopic Version: 一个生物体的各组成部分的行为能用计算机来模拟。也就是说,任何元素(为典型 起⻅,就假定是一个细胞)的行为,都能用一个 FlooP 程序(即一般递归函数)——在给定该元素的内部状态和外部环境的一个足够精确的描述之后——计算到任意精确的程度
- 简化理论形式:全部的大脑过程都可以从一个可计算的基质中导出
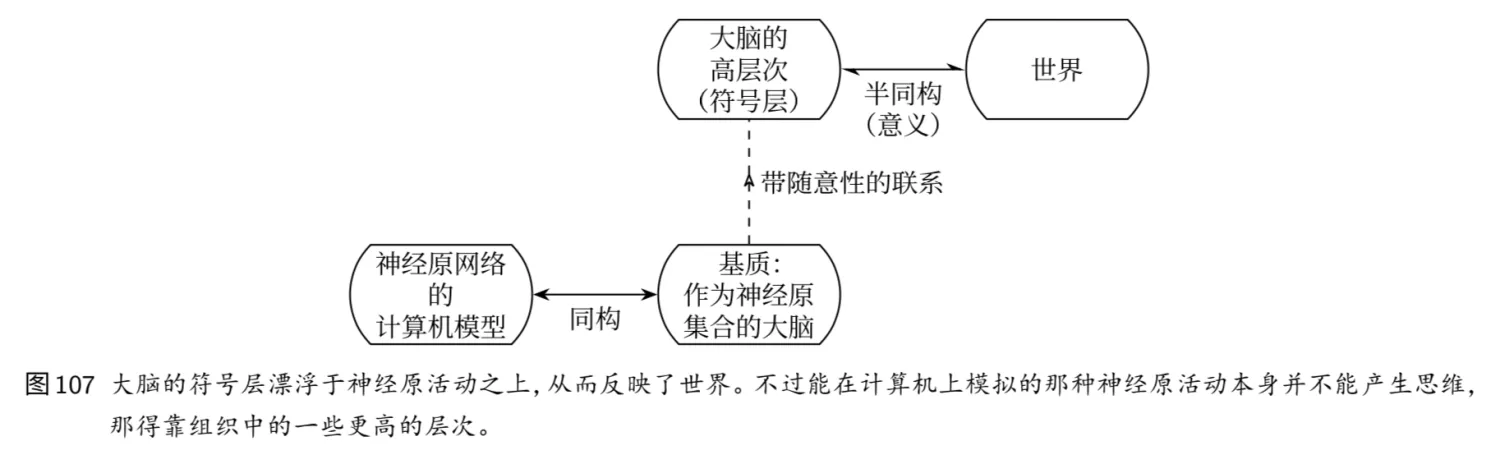
这是能支持“最终可能实现人工智能”这种观点的最强有力的理论基础,但是人工智能并不致力于模拟神经元网络,因为他建立在另一种信念之上,也就是说可能有一些意义重大的智能特征是漂浮在一些与生物大脑的基质完全不同的种类的基质之上的
reductionist faith, that all brain processes are derived from a computable subrate
this is the strongest theoretical underpinning that could support the eventual possibility of realizing Artificial Intelligence
AI resaerch is however not aimed at simulating neural networks, for it is based on another kind of faith, that probably there are significant features of intelligence that can be floated on top of enteirely different sorts of subrates thatn those of organic brains, figure 108
人工智能与大脑模拟的平行进展Parallel Progress in AI and Brain Simulation
有人认为,要想实现人工智能,就总有一天得要模拟或复制大脑的实际硬件。这种想法至少迄今为止是使 很多人工智能工作者极为反感的。人们仍在纳闷:“我们得把大脑模拟到什么精度才算实现了人工智能?”实际 答案可能是:这完全依赖于你想要模拟人类意识的多少特征。 如果只是玩跳棋或者做微积分,那么人工智能已经存在了。
有些时候,当 我们朝着人工智能方向前进了一步之后,却仿佛不是造出了某种大家都承认的确是智能的东西,而只是弄清了 。实际智能不是哪一种东西。如果智能包括学习、创造、情感响应、美的感受力、自我意识,那前面的路就还⻓,而且可能一直要到我们完全复制了一个活的大脑,才算是实现了这些 。
Each new step towards AI, rather than producing something which everyone agrees is real intelligence, merely reveals what real intelligence is not. if intelligence involves learning, creativity, emotional responses, a sense of beauty, a sense of self, then there is a long road ahead, and it may be that these will only be realized when we have totally duplicated a living brain
美感、螃蟹和灵魂Beauty, the Crab and the Soul
螃蟹在阿基里斯的大师级表演与这又有什么关系?Crab's virtuoso performance in front of Achilles
- 唯灵论形式Church-Turing Thesis, Soulist Version: 大脑所能做的某些种类的事情可以大致地由一台计算机来模拟,不过不是大多 数事情,而是些不那么吸引人的事情。不管怎么说,即使都能模拟,灵魂仍将留待解释,而且没有什么方法能让计算机来承担这个任务。 some kinds of things which a brain can do can be vaguely approximated on a computer but not most, and certainly not the interesting ones, but anywa, even if they all could, that would still leave the soul to explain, and there is no way that computers have any bearing on that
诸如美感是与难以琢磨的心灵相联的一种特性,声来就只能为人所有,单单机器是不行的
- 反科学形式 Theodore Roszak(美国counter-culture, ecopsychology发起人) Version: computers are ridiculous, so is science in general
the depth and complexty and beauty involved in explaining abstract structures such as the human mind, where in deed, one comes in intimate contact with the ultimate questions of what to be human is 在那些认为带有数量和严格性味道的东西是对人类价值的一种威胁的人们中间,这种观点颇为流行。真是 令人遗憾,他们意识不到深究诸如人类心智等抽象结构时所包含的深刻性、复杂性和美,正是在那里,需要密切 联系“人是什么”这样一个根本问题。
有的人认为没的鉴赏需要非理性的因素,因而与计算机的条理性不相容。
非理性与理性可以共存于不同的层次Irrational and Rational can Coexist on different levels
非理性与计算机不相容?层次混淆了
在一个层次上完美无缺的运行如何会支持较高层次上的符号操作——而高层上的目标却可能与真理的传播毫无关系。 Goals on higher level may be completely unrealted to the propagation of truth, thus symbolic manipulation of a higher level could instruct faultless functioning on one level
大脑是由一些运行得完美无缺的元素——神经元——所组成的集合体。 决不会有一个神经元忘记它的算术知识—— 把它的输入⻢⻢⻁⻁地加起来得到一个错误答案。即使一个神经元死了,它仍能在下述意义上正确行动:它的 组成元素继续遵从数学和物理的规律。而且,众所周知,神经元完全有可能在它自己的层次上以极其完美的支持错误的高层行为。没有什么理由使人相信,一台计算机中运转得完美无缺的硬件不可能支持那些体现诸如混乱、遗忘以及美 感这类复杂事件的高层符号行为。这将需要大量的子系统按照一种复杂的“逻辑”相互作用。表面的行为可能表现为理性或非理性,然而暗地里则应该是可靠的逻辑硬件的工作。
the brain is a collection of faultlessly functioning elements - neurons, a neuron does not forget its arithmetical knowledge , even when it dies, it continues to function correctly, yet they are perfectly able to support high level behavior that is wrong, on it on level
an incorrect belief held in the software of a mind, supoprted by the hardware of a fulatlessly functioning brain,on the lower level, brain is performing perfectly rational activities, faultless functioning, while brain appreciate beauty, and the irrationality is an epiphenomenon
overt behavior can be rational or irrational but undernearth it is reliable logical hardwared
再驳卢卡斯More Against Lucas
用层次区别来与卢卡斯辩论,人工智能的确是形式系统的实例,并且依赖于底层的硬件行动,但人工智能不是依赖于编程,由编程体现的。逻辑思维过程是人工智能的结果,而不是预先程序化了的东西。logical thought processes are consequesnces of the general intelligence of an AI program, rather than being programmed
人工智能的基点An Underpinning of AI
- 人工智能版本AI Version: 任何种类的心智过程都可以用一个计算机程序来模拟,而该程序的基础语言 与 FlooP 一样强,也就是说全体部分递归函数都能用这种语言程序化 mental processes of any sort can be simulated by a computer program whose underlying langauge is of power equal to that of FlooP, in which all partial recursive fucntions can be programmed
人工智能论题:随着智能机的发展,它的基础机制会逐渐收敛于人类智能的基础机制。
AI Thesis: as intelligence of machinese evolves, its underlying mechanisms will gradually converge to the mechanisms underlying human intelligence
人工智能工作者需要更多的深入到较低层次,使其越来越接近大脑的机制。on the lower levels to push closer and closer to brain mechanisms
丘奇定理Church's Theorem
there is an unsolvable paradox
Tarski's Theorem
no methods could tell theorems from nontheorems
被赞美螃蟹的不可能性The Impossibility of the Magnificrab
Crab's mind cannot be a truth-recognizer any more than it is a
TNT theorem recognizer
两种类型的形式Two types of Form
meanings
interpreters which accept two-dimensional patterns and then pull from them high-dimensional notions which are so complex that we cannot describe them
inner meaning reveals more of itself over a period of time,
- 句法方面form: well formedness, which can be detected by BlooP programs, syntactic qualities 可以用预知有终止的检验来检查
- 语义方面semantic aspects, cannot be tested and require open-ended tests , the object's meaning is not localized within the obejct itself. 不能在预定期限内检验,需要无尽头的检验,一个客体的意义并不局限于该客体自身之内。这自然并不是要说在时间终结之前就不可能理解任何 客体的意义。因为,随着时间的流逝,越来越多的意义变明朗了。但是,不论过多久,也不可能发现其意义的所有 方面。
意义来自认知结构的关系Meaning derives from Connections to Cognitive Structures
乐曲的意义有一种少⻅的两 重性:一方面,凭借着它与世界上其它很多事物的关系,似乎要向四周蔓延——而另一方面,一段乐曲的意义显 然源自音乐本身,所以它必定能定位于音乐内部的某个地方
The Interpreter音乐解释机制是通过建立一个多维的认知结构——该乐曲的一个心智表示——而工作的。 这个多维认知结构努力寻找与那些为以前的经验编码的多维心智结构之间的联系,借此同早就存在的信息结 合起来。随着这个过程的进行,整个意义就逐渐显露出来了。事实上,当一个人感到他已经悟透一段乐曲的核 心意义时,可能若干年的时间已经过去了。这似乎又有利于相反的观点:音乐的意义向四方延伸,解释机制的 作用就是逐步收集它。
multidiensional cognitive structure a mental representation of the piece, which it tries to integrate with pre-existente information by finding links with other multidimensional mental structures which encode previous experiences
the musical meaning is spread around ,the interpreter's role being to assemble it gradually
句法 syntactic: reside unambiguously inside the object under consideration, not hidden
语义 semantic: depends on its relationship with a potentially infinite class of other objects, and not completely localizable , hidden
句法性 质毫不含糊地存在于所考虑的客体之内,而语义性质则依赖于它与潜在地无穷多的客体类之间的关系,因而不 是完全可以定位的。原则上说,句法性质中没有什么秘密或隐藏的东西,然而这种隐蔽性却是语义性质的本质。 这就是我区别视觉形式的“句法”方面和“语义”方面的理由。
美、真与形式Beauty Truth and Form
various interpreter various aspect, beauty in Magnificrab with truth, is one of the most intangible notions
美的概念极难把握,所以和真的概念联系在一起,真乃是元数学里面最不好琢磨的概念之一
说谎者悖论的神经基质Neural Substrate of the Epimenides Paradox
Epimenides Paradox,
one level it is a sentence about itself which would be true if it is falce, arithetical substrate
abandoning the notion that the brain can provide fully accurate representation of the noint of truth
total modeling of truth is imporssible for quite physical reasons, it would require physically incompatble events to occure
说谎者悖论需要找到基质,和较低的互相冲突的物理时间,也就是按其性质而言不能同时出现的事件组成的层次。给出真理的全面模型是不可能的,因为做这样一个模型从物理上需要在一个大脑中出现不相容的两个事件。
联想,智慧还是分裂?
The test of a first-rate intelligence is the ability to hold two opposed ideas in the mind at the same time, and still retain the ability to function. — F. Scott Fitzgerald
Cognitive Dissonance: Mental stress or discomfort experienced by an individual who holds two or more contradictory beliefs, ideas, or values at the same time.